↑

Properties

Label 4-10e2-1.1-c23e2-0-1
Degree $4$
Conductor $100$
Sign $1$
Analytic cond. $1123.61$
Root an. cond. $5.78968$
Motivic weight $23$
Arithmetic yes
Rational yes
Primitive no
Self-dual yes
Analytic rank $2$

Origins

Origins of factors

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  − 4.09e3·2-s − 1.85e4·3-s + 1.25e7·4-s + 9.76e7·5-s + 7.58e7·6-s − 4.41e9·7-s − 3.43e10·8-s − 1.31e11·9-s − 4.00e11·10-s + 1.95e11·11-s − 2.32e11·12-s + 2.58e12·13-s + 1.80e13·14-s − 1.80e12·15-s + 8.79e13·16-s + 1.31e14·17-s + 5.36e14·18-s − 1.76e12·19-s + 1.22e15·20-s + 8.17e13·21-s − 7.98e14·22-s + 4.01e15·23-s + 6.36e14·24-s + 7.15e15·25-s − 1.05e16·26-s + 3.11e15·27-s − 5.55e16·28-s + ⋯
L(s)  = 1  − 1.41·2-s − 0.0603·3-s + 3/2·4-s + 0.894·5-s + 0.0853·6-s − 0.844·7-s − 1.41·8-s − 1.39·9-s − 1.26·10-s + 0.206·11-s − 0.0905·12-s + 0.399·13-s + 1.19·14-s − 0.0539·15-s + 5/4·16-s + 0.931·17-s + 1.96·18-s − 0.00346·19-s + 1.34·20-s + 0.0509·21-s − 0.291·22-s + 0.878·23-s + 0.0853·24-s + 3/5·25-s − 0.565·26-s + 0.107·27-s − 1.26·28-s + ⋯

Functional equation

\[\begin{aligned}\Lambda(s)=\mathstrut & 100 ^{s/2} \, \Gamma_{\C}(s)^{2} \, L(s)\cr =\mathstrut & \, \Lambda(24-s) \end{aligned}\]
\[\begin{aligned}\Lambda(s)=\mathstrut & 100 ^{s/2} \, \Gamma_{\C}(s+23/2)^{2} \, L(s)\cr =\mathstrut & \, \Lambda(1-s) \end{aligned}\]

Invariants

Degree: \(4\)
Conductor: \(100\)    =    \(2^{2} \cdot 5^{2}\)
Sign: $1$
Analytic conductor: \(1123.61\)
Root analytic conductor: \(5.78968\)
Motivic weight: \(23\)
Rational: yes
Arithmetic: yes
Character: Trivial
Primitive: no
Self-dual: yes
Analytic rank: \(2\)
Selberg data: \((4,\ 100,\ (\ :23/2, 23/2),\ 1)\)

Particular Values

\(L(12)\) \(=\) \(0\)
\(L(\frac12)\) \(=\) \(0\)
\(L(\frac{25}{2})\) not available
\(L(1)\) not available

Euler product

   \(L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1} \)
$p$$\Gal(F_p)$$F_p(T)$
bad2$C_1$ \( ( 1 + p^{11} T )^{2} \)
5$C_1$ \( ( 1 - p^{11} T )^{2} \)
good3$D_{4}$ \( 1 + 6172 p T + 540524626 p^{5} T^{2} + 6172 p^{24} T^{3} + p^{46} T^{4} \)
7$D_{4}$ \( 1 + 4415735108 T + 990635565626217198 p^{2} T^{2} + 4415735108 p^{23} T^{3} + p^{46} T^{4} \)
11$D_{4}$ \( 1 - 17728839984 p T + \)\(57\!\cdots\!86\)\( p^{2} T^{2} - 17728839984 p^{24} T^{3} + p^{46} T^{4} \)
13$D_{4}$ \( 1 - 2583183058684 T + \)\(54\!\cdots\!66\)\( p T^{2} - 2583183058684 p^{23} T^{3} + p^{46} T^{4} \)
17$D_{4}$ \( 1 - 7745227211796 p T + \)\(13\!\cdots\!38\)\( p^{2} T^{2} - 7745227211796 p^{24} T^{3} + p^{46} T^{4} \)
19$D_{4}$ \( 1 + 92678337800 p T - \)\(47\!\cdots\!62\)\( p^{2} T^{2} + 92678337800 p^{24} T^{3} + p^{46} T^{4} \)
23$D_{4}$ \( 1 - 4012836105342564 T + \)\(41\!\cdots\!58\)\( T^{2} - 4012836105342564 p^{23} T^{3} + p^{46} T^{4} \)
29$D_{4}$ \( 1 + 108385465473787380 T + \)\(11\!\cdots\!78\)\( T^{2} + 108385465473787380 p^{23} T^{3} + p^{46} T^{4} \)
31$D_{4}$ \( 1 + 282534241930647896 T + \)\(58\!\cdots\!86\)\( T^{2} + 282534241930647896 p^{23} T^{3} + p^{46} T^{4} \)
37$D_{4}$ \( 1 + 326855967548366468 T + \)\(23\!\cdots\!62\)\( T^{2} + 326855967548366468 p^{23} T^{3} + p^{46} T^{4} \)
41$D_{4}$ \( 1 + 5378411403014673276 T + \)\(31\!\cdots\!86\)\( T^{2} + 5378411403014673276 p^{23} T^{3} + p^{46} T^{4} \)
43$D_{4}$ \( 1 + 9824206470496391636 T + \)\(93\!\cdots\!38\)\( T^{2} + 9824206470496391636 p^{23} T^{3} + p^{46} T^{4} \)
47$D_{4}$ \( 1 + 18459008132084260308 T + \)\(56\!\cdots\!62\)\( T^{2} + 18459008132084260308 p^{23} T^{3} + p^{46} T^{4} \)
53$D_{4}$ \( 1 + 6735586459267392756 T + \)\(74\!\cdots\!38\)\( T^{2} + 6735586459267392756 p^{23} T^{3} + p^{46} T^{4} \)
59$D_{4}$ \( 1 - \)\(40\!\cdots\!40\)\( T + \)\(14\!\cdots\!58\)\( T^{2} - \)\(40\!\cdots\!40\)\( p^{23} T^{3} + p^{46} T^{4} \)
61$D_{4}$ \( 1 - \)\(26\!\cdots\!04\)\( T + \)\(10\!\cdots\!66\)\( T^{2} - \)\(26\!\cdots\!04\)\( p^{23} T^{3} + p^{46} T^{4} \)
67$D_{4}$ \( 1 + \)\(87\!\cdots\!48\)\( T + \)\(20\!\cdots\!02\)\( T^{2} + \)\(87\!\cdots\!48\)\( p^{23} T^{3} + p^{46} T^{4} \)
71$D_{4}$ \( 1 + \)\(14\!\cdots\!36\)\( T + \)\(80\!\cdots\!46\)\( T^{2} + \)\(14\!\cdots\!36\)\( p^{23} T^{3} + p^{46} T^{4} \)
73$D_{4}$ \( 1 + \)\(27\!\cdots\!76\)\( T + \)\(64\!\cdots\!78\)\( T^{2} + \)\(27\!\cdots\!76\)\( p^{23} T^{3} + p^{46} T^{4} \)
79$D_{4}$ \( 1 + \)\(16\!\cdots\!20\)\( T + \)\(19\!\cdots\!82\)\( p T^{2} + \)\(16\!\cdots\!20\)\( p^{23} T^{3} + p^{46} T^{4} \)
83$D_{4}$ \( 1 + \)\(24\!\cdots\!56\)\( T + \)\(38\!\cdots\!58\)\( T^{2} + \)\(24\!\cdots\!56\)\( p^{23} T^{3} + p^{46} T^{4} \)
89$D_{4}$ \( 1 + \)\(69\!\cdots\!80\)\( T + \)\(24\!\cdots\!38\)\( T^{2} + \)\(69\!\cdots\!80\)\( p^{23} T^{3} + p^{46} T^{4} \)
97$D_{4}$ \( 1 - \)\(14\!\cdots\!12\)\( T + \)\(12\!\cdots\!82\)\( T^{2} - \)\(14\!\cdots\!12\)\( p^{23} T^{3} + p^{46} T^{4} \)
show more
show less
   \(L(s) = \displaystyle\prod_p \ \prod_{j=1}^{4} (1 - \alpha_{j,p}\, p^{-s})^{-1}\)

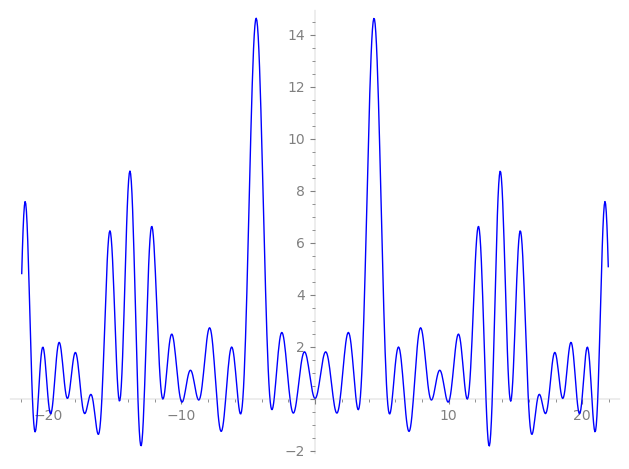
Imaginary part of the first few zeros on the critical line

−14.69390718322505392582060265338, −14.58169983205091940843880867931, −13.25939727512564272838476998147, −12.79049491196663800966999533364, −11.42728542323827566773725694286, −11.35150879129843082742683915823, −10.08346713543973632897355434531, −9.828036761207862126136814570754, −8.795024627342551685736053765080, −8.624538790419002043482374226643, −7.35627020794432526751101012849, −6.69201322082391387620331792881, −5.77645227090916990841145681726, −5.40606186739822459580152865245, −3.38027992499909036996118919657, −3.06197370869439677911210663828, −1.87873178612466061232391881201, −1.36549710130965868341011250098, 0, 0, 1.36549710130965868341011250098, 1.87873178612466061232391881201, 3.06197370869439677911210663828, 3.38027992499909036996118919657, 5.40606186739822459580152865245, 5.77645227090916990841145681726, 6.69201322082391387620331792881, 7.35627020794432526751101012849, 8.624538790419002043482374226643, 8.795024627342551685736053765080, 9.828036761207862126136814570754, 10.08346713543973632897355434531, 11.35150879129843082742683915823, 11.42728542323827566773725694286, 12.79049491196663800966999533364, 13.25939727512564272838476998147, 14.58169983205091940843880867931, 14.69390718322505392582060265338

Graph of the $Z$-function along the critical line