↑

Properties

Label 2-980-7.2-c1-0-5
Degree 22
Conductor 980980
Sign 0.386+0.922i0.386 + 0.922i
Analytic cond. 7.825337.82533
Root an. cond. 2.797382.79738
Motivic weight 11
Arithmetic yes
Rational no
Primitive yes
Self-dual no
Analytic rank 00

Origins

Related objects

Downloads

Learn more

Normalization:  

Dirichlet series

L(s)  = 1  + (−1.5 − 2.59i)3-s + (−0.5 + 0.866i)5-s + (−3 + 5.19i)9-s + (1 + 1.73i)11-s + 6·13-s + 3·15-s + (1 + 1.73i)17-s + (4.5 − 7.79i)23-s + (−0.499 − 0.866i)25-s + 9·27-s + 3·29-s + (1 + 1.73i)31-s + (3 − 5.19i)33-s + (−4 + 6.92i)37-s + (−9 − 15.5i)39-s + ⋯
L(s)  = 1  + (−0.866 − 1.49i)3-s + (−0.223 + 0.387i)5-s + (−1 + 1.73i)9-s + (0.301 + 0.522i)11-s + 1.66·13-s + 0.774·15-s + (0.242 + 0.420i)17-s + (0.938 − 1.62i)23-s + (−0.0999 − 0.173i)25-s + 1.73·27-s + 0.557·29-s + (0.179 + 0.311i)31-s + (0.522 − 0.904i)33-s + (−0.657 + 1.13i)37-s + (−1.44 − 2.49i)39-s + ⋯

Functional equation

Λ(s)=(980s/2 ΓC(s) L(s)=((0.386+0.922i) Λ‾(2−s)\begin{aligned}\Lambda(s)=\mathstrut & 980 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & (0.386 + 0.922i)\, \overline{\Lambda}(2-s) \end{aligned}
Λ(s)=(980s/2 ΓC(s+1/2) L(s)=((0.386+0.922i) Λ‾(1−s)\begin{aligned}\Lambda(s)=\mathstrut & 980 ^{s/2} \, \Gamma_{\C}(s+1/2) \, L(s)\cr =\mathstrut & (0.386 + 0.922i)\, \overline{\Lambda}(1-s) \end{aligned}

Invariants

Degree: 22
Conductor: 980980    =    22⋅5⋅722^{2} \cdot 5 \cdot 7^{2}
Sign: 0.386+0.922i0.386 + 0.922i
Analytic conductor: 7.825337.82533
Root analytic conductor: 2.797382.79738
Motivic weight: 11
Rational: no
Arithmetic: yes
Character: χ980(961,⋅)\chi_{980} (961, \cdot )
Primitive: yes
Self-dual: no
Analytic rank: 00
Selberg data: (2, 980, ( :1/2), 0.386+0.922i)(2,\ 980,\ (\ :1/2),\ 0.386 + 0.922i)

Particular Values

L(1)L(1) ≈\approx 0.986053−0.655905i0.986053 - 0.655905i
L(12)L(\frac12) ≈\approx 0.986053−0.655905i0.986053 - 0.655905i
L(32)L(\frac{3}{2}) not available
L(1)L(1) not available

Euler product

   L(s)=∏pFp(p−s)−1L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1}
ppFp(T)F_p(T)
bad2 1 1
5 1+(0.5−0.866i)T 1 + (0.5 - 0.866i)T
7 1 1
good3 1+(1.5+2.59i)T+(−1.5+2.59i)T2 1 + (1.5 + 2.59i)T + (-1.5 + 2.59i)T^{2}
11 1+(−1−1.73i)T+(−5.5+9.52i)T2 1 + (-1 - 1.73i)T + (-5.5 + 9.52i)T^{2}
13 1−6T+13T2 1 - 6T + 13T^{2}
17 1+(−1−1.73i)T+(−8.5+14.7i)T2 1 + (-1 - 1.73i)T + (-8.5 + 14.7i)T^{2}
19 1+(−9.5−16.4i)T2 1 + (-9.5 - 16.4i)T^{2}
23 1+(−4.5+7.79i)T+(−11.5−19.9i)T2 1 + (-4.5 + 7.79i)T + (-11.5 - 19.9i)T^{2}
29 1−3T+29T2 1 - 3T + 29T^{2}
31 1+(−1−1.73i)T+(−15.5+26.8i)T2 1 + (-1 - 1.73i)T + (-15.5 + 26.8i)T^{2}
37 1+(4−6.92i)T+(−18.5−32.0i)T2 1 + (4 - 6.92i)T + (-18.5 - 32.0i)T^{2}
41 1+5T+41T2 1 + 5T + 41T^{2}
43 1−T+43T2 1 - T + 43T^{2}
47 1+(−4+6.92i)T+(−23.5−40.7i)T2 1 + (-4 + 6.92i)T + (-23.5 - 40.7i)T^{2}
53 1+(2+3.46i)T+(−26.5+45.8i)T2 1 + (2 + 3.46i)T + (-26.5 + 45.8i)T^{2}
59 1+(4+6.92i)T+(−29.5+51.0i)T2 1 + (4 + 6.92i)T + (-29.5 + 51.0i)T^{2}
61 1+(−3.5+6.06i)T+(−30.5−52.8i)T2 1 + (-3.5 + 6.06i)T + (-30.5 - 52.8i)T^{2}
67 1+(−1.5−2.59i)T+(−33.5+58.0i)T2 1 + (-1.5 - 2.59i)T + (-33.5 + 58.0i)T^{2}
71 1−8T+71T2 1 - 8T + 71T^{2}
73 1+(−7−12.1i)T+(−36.5+63.2i)T2 1 + (-7 - 12.1i)T + (-36.5 + 63.2i)T^{2}
79 1+(2−3.46i)T+(−39.5−68.4i)T2 1 + (2 - 3.46i)T + (-39.5 - 68.4i)T^{2}
83 1−T+83T2 1 - T + 83T^{2}
89 1+(−6.5+11.2i)T+(−44.5−77.0i)T2 1 + (-6.5 + 11.2i)T + (-44.5 - 77.0i)T^{2}
97 1−10T+97T2 1 - 10T + 97T^{2}
show more
show less
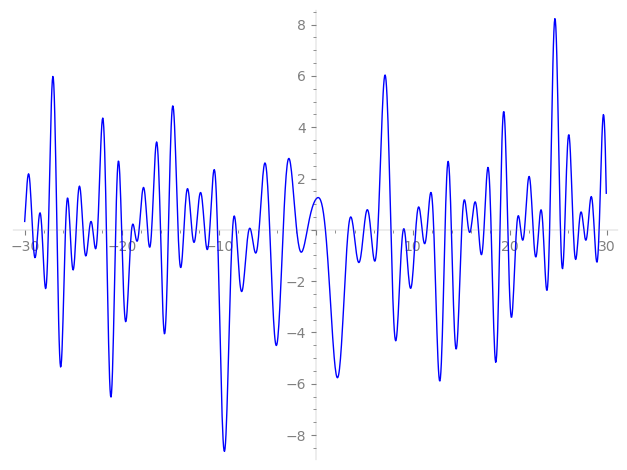
   L(s)=∏p ∏j=12(1−αj,p p−s)−1L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}

Imaginary part of the first few zeros on the critical line

−10.12415045780528937101596296056, −8.575985217166682750136144618897, −8.199973389632290018009885314443, −6.89064595664227616231617602403, −6.69752257954642342614077714698, −5.83866256231900707754218541761, −4.74011430270501850512274903563, −3.35323353790531445569869501298, −1.96222052862298600103783583546, −0.869566947810801854820645664028, 0.998071215017101942980472863605, 3.35284352103996439862655159452, 3.90327264761187513524774144251, 4.95869957540646618009965506755, 5.66851783944335284476522793276, 6.40432213967838869900548633656, 7.74886919339459238350470747174, 9.019429307288767411934948838560, 9.140461885245802917817875130940, 10.31129931620928935996612885302

Graph of the ZZ-function along the critical line