| L(s) = 1 | + 4·2-s + 10.4·3-s + 16·4-s + 95.5·5-s + 41.6·6-s + 232.·7-s + 64·8-s − 134.·9-s + 382.·10-s + 345.·11-s + 166.·12-s + 506.·13-s + 928.·14-s + 993.·15-s + 256·16-s − 797.·17-s − 539.·18-s + 1.52e3·20-s + 2.41e3·21-s + 1.38e3·22-s − 1.19e3·23-s + 665.·24-s + 6.00e3·25-s + 2.02e3·26-s − 3.92e3·27-s + 3.71e3·28-s − 425.·29-s + ⋯ |
| L(s) = 1 | + 0.707·2-s + 0.667·3-s + 0.5·4-s + 1.70·5-s + 0.471·6-s + 1.79·7-s + 0.353·8-s − 0.554·9-s + 1.20·10-s + 0.861·11-s + 0.333·12-s + 0.830·13-s + 1.26·14-s + 1.14·15-s + 0.250·16-s − 0.668·17-s − 0.392·18-s + 0.854·20-s + 1.19·21-s + 0.609·22-s − 0.469·23-s + 0.235·24-s + 1.92·25-s + 0.587·26-s − 1.03·27-s + 0.895·28-s − 0.0939·29-s + ⋯ |
\[\begin{aligned}\Lambda(s)=\mathstrut & 722 ^{s/2} \, \Gamma_{\C}(s) \, L(s)\cr =\mathstrut & \, \Lambda(6-s) \end{aligned}\]
\[\begin{aligned}\Lambda(s)=\mathstrut & 722 ^{s/2} \, \Gamma_{\C}(s+5/2) \, L(s)\cr =\mathstrut & \, \Lambda(1-s) \end{aligned}\]
Particular Values
| \(L(3)\) |
\(\approx\) |
\(8.448752276\) |
| \(L(\frac12)\) |
\(\approx\) |
\(8.448752276\) |
| \(L(\frac{7}{2})\) |
|
not available |
| \(L(1)\) |
|
not available |
\(L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1} \)
| $p$ | $F_p(T)$ |
|---|
| bad | 2 | \( 1 - 4T \) |
| 19 | \( 1 \) |
| good | 3 | \( 1 - 10.4T + 243T^{2} \) |
| 5 | \( 1 - 95.5T + 3.12e3T^{2} \) |
| 7 | \( 1 - 232.T + 1.68e4T^{2} \) |
| 11 | \( 1 - 345.T + 1.61e5T^{2} \) |
| 13 | \( 1 - 506.T + 3.71e5T^{2} \) |
| 17 | \( 1 + 797.T + 1.41e6T^{2} \) |
| 23 | \( 1 + 1.19e3T + 6.43e6T^{2} \) |
| 29 | \( 1 + 425.T + 2.05e7T^{2} \) |
| 31 | \( 1 - 6.64e3T + 2.86e7T^{2} \) |
| 37 | \( 1 + 7.85e3T + 6.93e7T^{2} \) |
| 41 | \( 1 + 1.60e4T + 1.15e8T^{2} \) |
| 43 | \( 1 - 1.29e4T + 1.47e8T^{2} \) |
| 47 | \( 1 + 2.11e4T + 2.29e8T^{2} \) |
| 53 | \( 1 + 2.90e4T + 4.18e8T^{2} \) |
| 59 | \( 1 - 9.34e3T + 7.14e8T^{2} \) |
| 61 | \( 1 + 8.32e3T + 8.44e8T^{2} \) |
| 67 | \( 1 + 1.43e4T + 1.35e9T^{2} \) |
| 71 | \( 1 + 3.12e4T + 1.80e9T^{2} \) |
| 73 | \( 1 - 1.99e3T + 2.07e9T^{2} \) |
| 79 | \( 1 + 9.62e3T + 3.07e9T^{2} \) |
| 83 | \( 1 + 3.41e4T + 3.93e9T^{2} \) |
| 89 | \( 1 + 1.11e5T + 5.58e9T^{2} \) |
| 97 | \( 1 - 9.69e4T + 8.58e9T^{2} \) |
| show more | |
| show less | |
\(L(s) = \displaystyle\prod_p \ \prod_{j=1}^{2} (1 - \alpha_{j,p}\, p^{-s})^{-1}\)
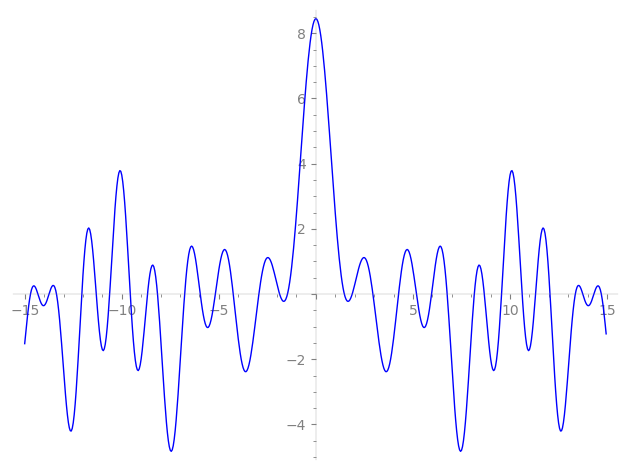
Imaginary part of the first few zeros on the critical line
−9.562616776769541336428071922162, −8.673879410028882314524417555570, −8.158700149558010807304842655887, −6.76070212616305520631692105410, −5.95730270231127897908512118386, −5.18011175765873910801690287494, −4.25746705066364843742463062073, −2.93674806577453306614705919880, −1.86934009788754607079442260520, −1.46439838065514260977292864376,
1.46439838065514260977292864376, 1.86934009788754607079442260520, 2.93674806577453306614705919880, 4.25746705066364843742463062073, 5.18011175765873910801690287494, 5.95730270231127897908512118386, 6.76070212616305520631692105410, 8.158700149558010807304842655887, 8.673879410028882314524417555570, 9.562616776769541336428071922162