| L(s) = 1 | − 4-s + 9-s + 16-s − 4·31-s − 36-s + 49-s − 64-s + 2·71-s − 2·109-s + 2·121-s + 4·124-s + 127-s + 131-s + 137-s + 139-s + 144-s + 149-s + 151-s + 157-s + 163-s + 167-s − 169-s + 173-s + 179-s + 181-s + 191-s + 193-s + ⋯ |
| L(s) = 1 | − 4-s + 9-s + 16-s − 4·31-s − 36-s + 49-s − 64-s + 2·71-s − 2·109-s + 2·121-s + 4·124-s + 127-s + 131-s + 137-s + 139-s + 144-s + 149-s + 151-s + 157-s + 163-s + 167-s − 169-s + 173-s + 179-s + 181-s + 191-s + 193-s + ⋯ |
\[\begin{aligned}\Lambda(s)=\mathstrut & 6760000 ^{s/2} \, \Gamma_{\C}(s)^{2} \, L(s)\cr =\mathstrut & \, \Lambda(1-s) \end{aligned}\]
\[\begin{aligned}\Lambda(s)=\mathstrut & 6760000 ^{s/2} \, \Gamma_{\C}(s)^{2} \, L(s)\cr =\mathstrut & \, \Lambda(1-s) \end{aligned}\]
Particular Values
| \(L(\frac{1}{2})\) |
\(\approx\) |
\(0.9382726912\) |
| \(L(\frac12)\) |
\(\approx\) |
\(0.9382726912\) |
| \(L(1)\) |
|
not available |
| \(L(1)\) |
|
not available |
\(L(s) = \displaystyle \prod_{p} F_p(p^{-s})^{-1} \)
| $p$ | $\Gal(F_p)$ | $F_p(T)$ |
|---|
| bad | 2 | $C_2$ | \( 1 + T^{2} \) |
| 5 | | \( 1 \) |
| 13 | $C_2$ | \( 1 + T^{2} \) |
| good | 3 | $C_2^2$ | \( 1 - T^{2} + T^{4} \) |
| 7 | $C_2^2$ | \( 1 - T^{2} + T^{4} \) |
| 11 | $C_1$$\times$$C_1$ | \( ( 1 - T )^{2}( 1 + T )^{2} \) |
| 17 | $C_2^2$ | \( 1 - T^{2} + T^{4} \) |
| 19 | $C_1$$\times$$C_1$ | \( ( 1 - T )^{2}( 1 + T )^{2} \) |
| 23 | $C_2$ | \( ( 1 + T^{2} )^{2} \) |
| 29 | $C_1$$\times$$C_1$ | \( ( 1 - T )^{2}( 1 + T )^{2} \) |
| 31 | $C_1$ | \( ( 1 + T )^{4} \) |
| 37 | $C_2^2$ | \( 1 - T^{2} + T^{4} \) |
| 41 | $C_1$$\times$$C_1$ | \( ( 1 - T )^{2}( 1 + T )^{2} \) |
| 43 | $C_2^2$ | \( 1 - T^{2} + T^{4} \) |
| 47 | $C_2^2$ | \( 1 - T^{2} + T^{4} \) |
| 53 | $C_2$ | \( ( 1 + T^{2} )^{2} \) |
| 59 | $C_1$$\times$$C_1$ | \( ( 1 - T )^{2}( 1 + T )^{2} \) |
| 61 | $C_1$$\times$$C_1$ | \( ( 1 - T )^{2}( 1 + T )^{2} \) |
| 67 | $C_2$ | \( ( 1 + T^{2} )^{2} \) |
| 71 | $C_2$ | \( ( 1 - T + T^{2} )^{2} \) |
| 73 | $C_2$ | \( ( 1 + T^{2} )^{2} \) |
| 79 | $C_1$$\times$$C_1$ | \( ( 1 - T )^{2}( 1 + T )^{2} \) |
| 83 | $C_2$ | \( ( 1 + T^{2} )^{2} \) |
| 89 | $C_1$$\times$$C_1$ | \( ( 1 - T )^{2}( 1 + T )^{2} \) |
| 97 | $C_2$ | \( ( 1 + T^{2} )^{2} \) |
| show more | | |
| show less | | |
\(L(s) = \displaystyle\prod_p \ \prod_{j=1}^{4} (1 - \alpha_{j,p}\, p^{-s})^{-1}\)
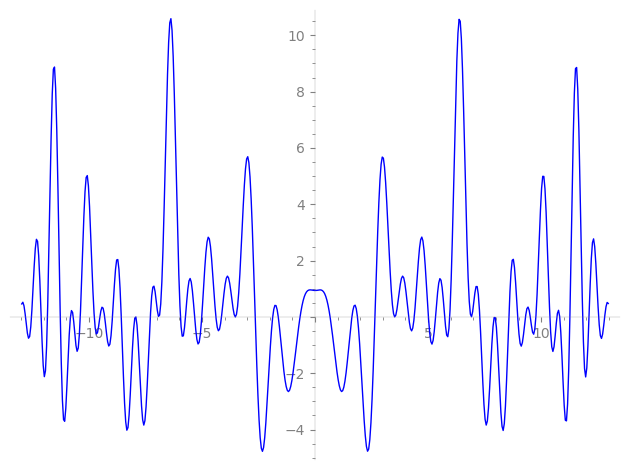
Imaginary part of the first few zeros on the critical line
−9.309858786027913465307337564615, −8.965143348731152654901595631742, −8.575401925430477705499808454035, −7.962780757441523473655793668422, −7.929221259602073935583366387689, −7.28887371156706214148821550113, −6.91302117050544894852730875095, −6.90802042933717720255091797914, −5.95533086980217124120116354545, −5.73485165838452192604891428599, −5.32910483653826253440168043965, −5.01437435126731726376976657464, −4.38710127369997047439325810473, −4.14127237860610942799375375590, −3.53339647873019745774335370303, −3.52232375631695779717175110278, −2.64874601056027250164842693353, −1.87403535638343678969713244945, −1.62874756083702419636601617607, −0.66400420368426980752917054715,
0.66400420368426980752917054715, 1.62874756083702419636601617607, 1.87403535638343678969713244945, 2.64874601056027250164842693353, 3.52232375631695779717175110278, 3.53339647873019745774335370303, 4.14127237860610942799375375590, 4.38710127369997047439325810473, 5.01437435126731726376976657464, 5.32910483653826253440168043965, 5.73485165838452192604891428599, 5.95533086980217124120116354545, 6.90802042933717720255091797914, 6.91302117050544894852730875095, 7.28887371156706214148821550113, 7.929221259602073935583366387689, 7.962780757441523473655793668422, 8.575401925430477705499808454035, 8.965143348731152654901595631742, 9.309858786027913465307337564615